Matrix manipulation
/**
* Copyright (c) 2003, www.pdfbox.org
* All rights reserved.
*
* Redistribution and use in source and binary forms, with or without
* modification, are permitted provided that the following conditions are met:
*
* 1. Redistributions of source code must retain the above copyright notice,
* this list of conditions and the following disclaimer.
* 2. Redistributions in binary form must reproduce the above copyright notice,
* this list of conditions and the following disclaimer in the documentation
* and/or other materials provided with the distribution.
* 3. Neither the name of pdfbox; nor the names of its
* contributors may be used to endorse or promote products derived from this
* software without specific prior written permission.
*
* THIS SOFTWARE IS PROVIDED BY THE COPYRIGHT HOLDERS AND CONTRIBUTORS "AS IS"
* AND ANY EXPRESS OR IMPLIED WARRANTIES, INCLUDING, BUT NOT LIMITED TO, THE
* IMPLIED WARRANTIES OF MERCHANTABILITY AND FITNESS FOR A PARTICULAR PURPOSE ARE
* DISCLAIMED. IN NO EVENT SHALL THE REGENTS OR CONTRIBUTORS BE LIABLE FOR ANY
* DIRECT, INDIRECT, INCIDENTAL, SPECIAL, EXEMPLARY, OR CONSEQUENTIAL DAMAGES
* (INCLUDING, BUT NOT LIMITED TO, PROCUREMENT OF SUBSTITUTE GOODS OR SERVICES;
* LOSS OF USE, DATA, OR PROFITS; OR BUSINESS INTERRUPTION) HOWEVER CAUSED AND ON
* ANY THEORY OF LIABILITY, WHETHER IN CONTRACT, STRICT LIABILITY, OR TORT
* (INCLUDING NEGLIGENCE OR OTHERWISE) ARISING IN ANY WAY OUT OF THE USE OF THIS
* SOFTWARE, EVEN IF ADVISED OF THE POSSIBILITY OF SUCH DAMAGE.
*
* http://www.pdfbox.org
*
*/
import java.awt.geom.AffineTransform;
/**
* This class will be used for matrix manipulation.
*
* @author <a href="mailto:ben@benlitchfield.com">Ben Litchfield</a>
* @version $Revision: 1.14 $
*/
public class Matrix implements Cloneable
{
private float[] single =
{
1,0,0,
0,1,0,
0,0,1
};
/**
* Constructor.
*/
public Matrix()
{
//default constructor
}
/**
* Create an affine transform from this matrix's values.
*
* @return An affine transform with this matrix's values.
*/
public AffineTransform createAffineTransform()
{
AffineTransform retval = new AffineTransform(
single[0], single[1],
single[3], single[4],
single[6], single[7] );
return retval;
}
/**
* Set the values of the matrix from the AffineTransform.
*
* @param af The transform to get the values from.
*/
public void setFromAffineTransform( AffineTransform af )
{
single[0] = (float)af.getScaleX();
single[1] = (float)af.getShearY();
single[3] = (float)af.getShearX();
single[4] = (float)af.getScaleY();
single[6] = (float)af.getTranslateX();
single[7] = (float)af.getTranslateY();
}
/**
* This will get a matrix value at some point.
*
* @param row The row to get the value from.
* @param column The column to get the value from.
*
* @return The value at the row/column position.
*/
public float getValue( int row, int column )
{
return single[row*3+column];
}
/**
* This will set a value at a position.
*
* @param row The row to set the value at.
* @param column the column to set the value at.
* @param value The value to set at the position.
*/
public void setValue( int row, int column, float value )
{
single[row*3+column] = value;
}
/**
* Return a single dimension array of all values in the matrix.
*
* @return The values ot this matrix.
*/
public float[][] getValues()
{
float[][] retval = new float[3][3];
retval[0][0] = single[0];
retval[0][1] = single[1];
retval[0][2] = single[2];
retval[1][0] = single[3];
retval[1][1] = single[4];
retval[1][2] = single[5];
retval[2][0] = single[6];
retval[2][1] = single[7];
retval[2][2] = single[8];
return retval;
}
/**
* Return a single dimension array of all values in the matrix.
*
* @return The values ot this matrix.
*/
public double[][] getValuesAsDouble()
{
double[][] retval = new double[3][3];
retval[0][0] = single[0];
retval[0][1] = single[1];
retval[0][2] = single[2];
retval[1][0] = single[3];
retval[1][1] = single[4];
retval[1][2] = single[5];
retval[2][0] = single[6];
retval[2][1] = single[7];
retval[2][2] = single[8];
return retval;
}
/**
* This will take the current matrix and multipy it with a matrix that is passed in.
*
* @param b The matrix to multiply by.
*
* @return The result of the two multiplied matrices.
*/
public Matrix multiply( Matrix b )
{
Matrix result = new Matrix();
float[] bMatrix = b.single;
float[] resultMatrix = result.single;
resultMatrix[0] = single[0] * bMatrix[0] + single[1] * bMatrix[3] + single[2] * bMatrix[6];
resultMatrix[1] = single[0] * bMatrix[1] + single[1] * bMatrix[4] + single[2] * bMatrix[7];
resultMatrix[2] = single[0] * bMatrix[2] + single[1] * bMatrix[5] + single[2] * bMatrix[8];
resultMatrix[3] = single[3] * bMatrix[0] + single[4] * bMatrix[3] + single[5] * bMatrix[6];
resultMatrix[4] = single[3] * bMatrix[1] + single[4] * bMatrix[4] + single[5] * bMatrix[7];
resultMatrix[5] = single[3] * bMatrix[2] + single[4] * bMatrix[5] + single[5] * bMatrix[8];
resultMatrix[6] = single[6] * bMatrix[0] + single[7] * bMatrix[3] + single[8] * bMatrix[6];
resultMatrix[7] = single[6] * bMatrix[1] + single[7] * bMatrix[4] + single[8] * bMatrix[7];
resultMatrix[8] = single[6] * bMatrix[2] + single[7] * bMatrix[5] + single[8] * bMatrix[8];
return result;
}
/**
* Create a new matrix with just the scaling operators.
*
* @return A new matrix with just the scaling operators.
*/
public Matrix extractScaling()
{
Matrix retval = new Matrix();
retval.single[0] = this.single[0];
retval.single[4] = this.single[4];
return retval;
}
/**
* Convenience method to create a scaled instance.
*
* @param x The xscale operator.
* @param y The yscale operator.
* @return A new matrix with just the x/y scaling
*/
public static Matrix getScaleInstance( float x, float y)
{
Matrix retval = new Matrix();
retval.single[0] = x;
retval.single[4] = y;
return retval;
}
/**
* Create a new matrix with just the translating operators.
*
* @return A new matrix with just the translating operators.
*/
public Matrix extractTranslating()
{
Matrix retval = new Matrix();
retval.single[6] = this.single[6];
retval.single[7] = this.single[7];
return retval;
}
/**
* Convenience method to create a translating instance.
*
* @param x The x translating operator.
* @param y The y translating operator.
* @return A new matrix with just the x/y translating.
*/
public static Matrix getTranslatingInstance( float x, float y)
{
Matrix retval = new Matrix();
retval.single[6] = x;
retval.single[7] = y;
return retval;
}
/**
* Clones this object.
* @return cloned matrix as an object.
*/
public Object clone()
{
Matrix clone = new Matrix();
System.arraycopy( single, 0, clone.single, 0, 9 );
return clone;
}
/**
* This will copy the text matrix data.
*
* @return a matrix that matches this one.
*/
public Matrix copy()
{
return (Matrix) clone();
}
/**
* This will return a string representation of the matrix.
*
* @return The matrix as a string.
*/
public String toString()
{
StringBuffer result = new StringBuffer( "" );
result.append( "[[" );
result.append( single[0] + "," );
result.append( single[1] + "," );
result.append( single[2] + "][");
result.append( single[3] + "," );
result.append( single[4] + "," );
result.append( single[5] + "][");
result.append( single[6] + "," );
result.append( single[7] + "," );
result.append( single[8] + "]]");
return result.toString();
}
/**
* Get the xscaling factor of this matrix.
* @return The x-scale.
*/
public float getXScale()
{
float xScale = single[0];
/**
* BM: if the trm is rotated, the calculation is a little more complicated
*
* The rotation matrix multiplied with the scaling matrix is:
* ( x 0 0) ( cos sin 0) ( x*cos x*sin 0)
* ( 0 y 0) * (-sin cos 0) = (-y*sin y*cos 0)
* ( 0 0 1) ( 0 0 1) ( 0 0 1)
*
* So, if you want to deduce x from the matrix you take
* M(0,0) = x*cos and M(0,1) = x*sin and use the theorem of Pythagoras
*
* sqrt(M(0,0)^2+M(0,1)^2) =
* sqrt(x2*cos2+x2*sin2) =
* sqrt(x2*(cos2+sin2)) = <- here is the trick cos2+sin2 is one
* sqrt(x2) =
* abs(x)
*/
if( !(single[1]==0.0f && single[3]==0.0f) )
{
xScale = (float)Math.sqrt(Math.pow(single[0], 2)+
Math.pow(single[1], 2));
}
return xScale;
}
/**
* Get the y scaling factor of this matrix.
* @return The y-scale factor.
*/
public float getYScale()
{
float yScale = single[4];
if( !(single[1]==0.0f && single[3]==0.0f) )
{
yScale = (float)Math.sqrt(Math.pow(single[3], 2)+
Math.pow(single[4], 2));
}
return yScale;
}
/**
* Get the x position in the matrix.
* @return The x-position.
*/
public float getXPosition()
{
return single[6];
}
/**
* Get the y position.
* @return The y position.
*/
public float getYPosition()
{
return single[7];
}
}
Related examples in the same category
| 1. | Absolute value | | |
| 2. | Find absolute value of float, int, double and long using Math.abs | | |
| 3. | Find ceiling value of a number using Math.ceil | | |
| 4. | Find exponential value of a number using Math.exp | | |
| 5. | Find floor value of a number using Math.floor | | |
| 6. | Find minimum of two numbers using Math.min | | |
| 7. | Find power using Math.pow | | |
| 8. | Find square root of a number using Math.sqrt | | |
| 9. | Find natural logarithm value of a number using Math.log | | |
| 10. | Find maximum of two numbers using Math.max | | |
| 11. | Get the power value | |  |
| 12. | Using the Math Trig Methods | | 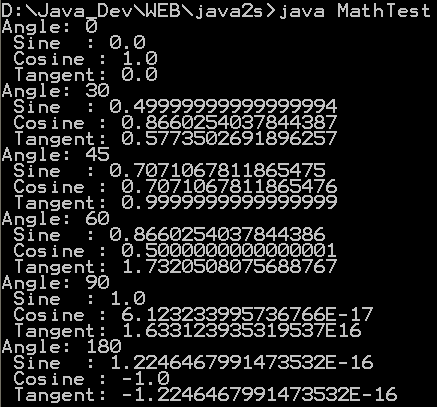 |
| 13. | Using BigDecimal for Precision | | 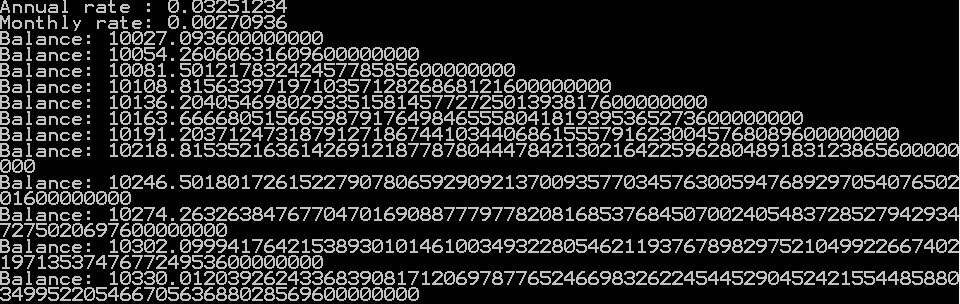 |
| 14. | Demonstrate our own version round() | | 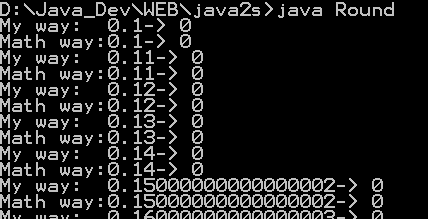 |
| 15. | Demonstrate a few of the Math functions for Trigonometry | | 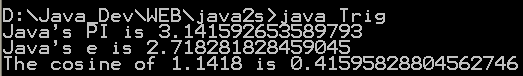 |
| 16. | Exponential Demo | |  |
| 17. | Min Demo | | |
| 18. | Basic Math Demo | | 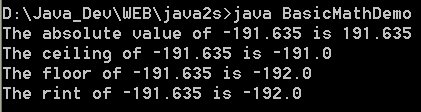 |
| 19. | Using strict math in applications | | 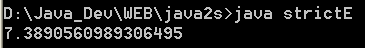 |
| 20. | Conversion between polar and rectangular coordinates | | |
| 21. | Using the pow() function | | |
| 22. | Using strict math at the method level | | |
| 23. | Calculating hyperbolic functions | | |
| 24. | Calculating trigonometric functions | | |
| 25. | Weighted floating-point comparisons | | |
| 26. | Solving right triangles | | |
| 27. | Applying the quadratic formula | | |
| 28. | Calculate the floor of the log, base 2 | | |
| 29. | Greatest Common Divisor (GCD) of positive integer numbers | | |
| 30. | Least Common Multiple (LCM) of two strictly positive integer numbers | | |
| 31. | Moving Average | | |
| 32. | Make Exponention | | |
| 33. | Caclulate the factorial of N | | |
| 34. | Trigonometric Demo | | 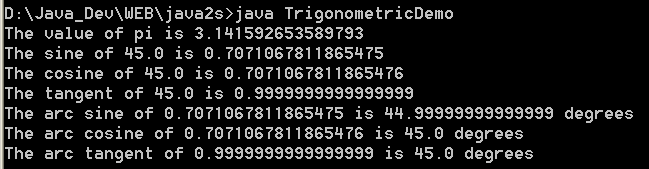 |
| 35. | Complex Number Demo | | |
| 36. | sqrt(a^2 + b^2) without under/overflow | | |
| 37. | Returns an integer hash code representing the given double array value. | | |
| 38. | Returns an integer hash code representing the given double value. | | |
| 39. | Returns n!. Shorthand for n Factorial, the product of the numbers 1,...,n as a double. | | |
| 40. | Returns n!. Shorthand for n Factorial, the product of the numbers 1,...,n. | | |
| 41. | Returns the hyperbolic sine of x. | | |
| 42. | Contains static definition for matrix math methods. | | |
| 43. | For a double precision value x, this method returns +1.0 if x >= 0 and -1.0 if x < 0. Returns NaN if x is NaN. | | |
| 44. | For a float value x, this method returns +1.0F if x >= 0 and -1.0F if x < 0. Returns NaN if x is NaN. | | |
| 45. | Normalize an angle in a 2&pi wide interval around a center value. | | |
| 46. | Normalizes an angle to a relative angle. | | |
| 47. | Normalizes an angle to an absolute angle | | |
| 48. | Normalizes an angle to be near an absolute angle | | |
| 49. | Returns the natural logarithm of n!. | | |
| 50. | Returns the least common multiple between two integer values. | | |
| 51. | Gets the greatest common divisor of the absolute value of two numbers | | |
| 52. | Returns exact (http://mathworld.wolfram.com/BinomialCoefficient.html) Binomial Coefficient | | |
| 53. | Returns a double representation of the (http://mathworld.wolfram.com/BinomialCoefficient.html) Binomial Coefficient | | |
| 54. | Returns the natural log of the (http://mathworld.wolfram.com/BinomialCoefficient.html) Binomial Coefficient | | |
| 55. | Returns the hyperbolic cosine of x. | | |
| 56. | Math Utils | | |
| 57. | Implements the methods which are in the standard J2SE's Math class, but are not in in J2ME's. | | |
| 58. | Utility methods for mathematical problems. | | |
| 59. | A math utility class with static methods. | | |
| 60. | Computes the binomial coefficient "n over k" | | |
| 61. | Log Gamma | | |
| 62. | Log Beta | | |
| 63. | Beta | | |
| 64. | Gamma | | |
| 65. | Factorial | | |
| 66. | Computes p(x;n,p) where x~B(n,p) | | |
| 67. | Returns the sum of two doubles expressed in log space | | |
| 68. | sigmod | | |
| 69. | sigmod rev | | |
| 70. | Numbers that are closer than this are considered equal | | |
| 71. | Returns the KL divergence, K(p1 || p2). | | |
| 72. | Returns the sum of two doubles expressed in log space | | |
| 73. | Returns the difference of two doubles expressed in log space | | |
| 74. | Is Prime | | |
| 75. | Statistical functions on arrays of numbers, namely, the mean, variance, standard deviation, covariance, min and max | | |
| 76. | This class calculates the Factorial of a numbers passed into the program through command line arguments. | |  |
| 77. | Calculates the Greatest Common Divisor of two numbers passed into the program through command line arguments. | | |
| 78. | Variance: the square of the standard deviation. | | |
| 79. | Population Standard Deviation | | |
| 80. | Returns from a static prime table the least prime that is greater than or equal to a specified value. | | |